DWI Pulse Sequence

How do you make a DW image?

Modern diffusion-weighted (DW) sequences all trace their origin to the pulsed gradient spin echo (PGSE) technique developed by Edward Stejskal and John Tanner in the mid-1960's. As shown in the diagram right, symmetric, strong diffusion-sensitizing gradients (DG's) are applied on either side of the 180°-pulse. The phases of stationary spins are unaffected by the DG pair since any phase accumulation from the first gradient lobe is reversed by the second. Diffusing spins, however, move into different locations between the first and second lobes, falling out of phase and losing signal. 

The Stejskal-Tanner pulsed gradient spin echo technique forms the basis of current diffusion-weighted pulse sequences. Stationary spins are not affected by the paired gradients, but diffusing spins are dephased.

Immediately following the second DG, an image acquisition module is played out. This is typically an echo-planar sequence using rapidly oscillating phase and frequency gradients that generate multiple gradient echoes. Rapid image acquisition is generally required to minimize the effects of bulk motion (such as vascular pulsations) on the DW images. Other modules (such as fast spin echo) are possible, but are not as widely used at the present time. 

Modern implementations of DWI retain the basic features of Stejskal's and Tanner's original PGSE technique with certain modifications. To suppress chemical shift artifacts, all commercial DWI sequences utilize some sort of fat suppression method. This may be a chemically-selective fat saturation pulse or a nonselective "STIR-like" inverting pulse applied immediately before the 90°-pulse.  Alternatively, the 90°-pulse itself may be selectively tuned to excite water protons only. To suppress eddy currents and reduce spatial distortion artifacts a "twice-refocused" PGSE sequence may be used. This technique employs a second 180°-refocusing pulse just before the image acquisition module begins. A third common modification to reduce eddy current artifacts involves the use of bipolar (rather than unipolar) DG's.

With the core pulse sequence defined as above, the following steps are automatically performed to generate DW images and their associated maps:

1. The DW pulse sequence is first run with the DG's turned off or set to a very low value. This generates a set of b0 ("b-zero") images that are T2-weighted and will serve as a baseline for later calculated maps.  (For abdominal imaging b50 images are often obtained, the small but nonzero gradient amplitude helping to suppress signal in vessels).
2. The DW sequence is then run with the DG's turned on individually or in combination and at various strengths. This produces DW source images sensitized to diffusion in multiple different directions.
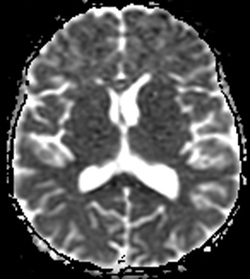
3. The DW source images are combined to produce a set of Trace DW images, the first-line images used for clinical diagnosis.
4. An Apparent Diffusion Coefficient (ADC) map is then calculated using the data from the the b0 and source images. The ADC map is used to clarify abnormalities seen on the trace images.
   
5. Further advanced processing can be optionally performed, creating additional calculated image sets for analysis. These may include exponential ADC maps, fractional anisotropy images, principal diffusion direction maps, and fiber tracking maps.

Examples of these various image sets are shown below and will be discussed more completely in subsequent Q&A's.

b0 image

Source DW image

Trace DW Image

ADC map

Exponential ADC map

Fractional Anisotropy map

Principal Diffusion Direction map

Fiber-tracking map

Advanced Discussion (show/hide)»

Most current commercial diffusion-weighted and diffusion tensor imaging sequences are based on single- or multi-shot echo planar imaging (EPI). The EPI acquisition mode results in the major artifacts seen in DW imaging (susceptibility and Nyquist ghosts, discussed in the artifacts section). As such, several alternative DWI and DTI strategies have been used to produce better quality images.

One commonly used method on several clinical platforms is to change the k-space trajectory from rectilinear (Cartesian) sampling to an overlapping radial method (PROPELLER/BLADE). By oversampling the center of k-space overall image contrast is improved and motion/bulk flow artifacts are minimized.

The use thinner slices with parallel imaging is also helpful to reduce susceptibility artifacts and is an option on several commercial MR systems.

A promising future method is to add diffusion gradients (DG's) into fast spin-echo (FSE) acquisitions. This is technically challenging in that incorporating DG's violates Carr-Purcell-Meiboom-Gill conditions* resulting in phase errors, signal loss, and artifacts due to inconsistent refocusing of the primary spin and stimulated echoes (STE's). Various strategies to overcome these limitations of FSE-DWI include:

• magnetization preparation;
• use of quadratic phase refocusing pulses;
• elimination of STEs by crusher gradients;
• splitting of the echo train to collect the SE's and STE's separately;
• use of navigator echoes to detect and correct phase errors due to motion; and
• use of a stimulated echo acquisition mode (STEAM) pulse sequence.

Because of the critical importance of DWI in modern clinical diagnosis, you can expect to see major advances in this area within the next few years.

----------------------------------

*The Carr-Purcell-Meiboom-Gill (CPMG) conditions are:

1. Refocusing pulses must be 90° out of phase with respect to the excitation pulse;
2. Spacing between pulses must be even and equal to twice the interval between the excitation and first refocusing pulse; and
3. The phases accumulated by spin isochromats between any two consecutive refocusing pulses must be equal.

When these conditions are met, the primary SE and STEs occur exactly at the midpoint between consecutive refocusing pulses and have the same phase.

---

References 
     Alexander AL, Tsuruda JS, Parker DL. Elimination of eddy current artifacts in diffusion-weighted echo-planar images: the use of bipolar gradients. Magn Reson Med 1997; 38:1016–21.
     Dietrich O, Biffar A, Baur-Melnyk A, Reiser MF. Technical aspects of diffusion imaging of the body. Eur J Radiol 2010; 76:314-322.
     Le Bihan D, Breton E, Lallemand D, et al. MR imaging of intravoxel incoherent motions: applications to diffusion and perfusion in neurologic disorders. Radiology 1986; 161:401-407.
     Minati L, Weglarz WP. Physical foundations, models, and methods of diffusion magnetic resonance imaging of the brain: a review. Concepts Magn Reson 2007; 30A:278-307.
     Mukherjee P, Berman JI, Chung SW, et al. Diffusion tensor MR imaging and fiber tractography: theoretic underpinnings. AJNR Am J Neuroradiol 2008; 29:632-640.
     Reese TG, Heid O, Weisskoff RM, Wedeen VJ. Reduction of eddy-current-induced distortion in diffusion MRI using a twice-refocused spin echo. Magn Reson Med 2003; 49:177-182. (Explains why adding a second 180°-pulse reduces eddy currents).
     Sinnaeve D. The Stejskal–Tanner equation generalized for any gradient shape—an overview of most pulse sequences measuring free diffusion. Concepts Magn Reson Part A 2012; 40A:39-65.
     Stejskal EO, Tanner JE. Spin diffusion measurements: spin echoes in the presence of
time-dependent field gradient. J Chem Phys 1965; 42:288-292. (This is the "classic").
     Tournier J-D, Mori S, Leemans A. Diffusion tensor imaging and beyond. Magn Reson Med 2011; 65:1532-1556.

---

Related Questions
     What is diffusion?
     Which diseases are "bright" on DW imaging and why?  

---

←  Previous Question

Next Question  →

↑ Complete List of Questions ↑